
(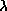 is the wavelength, and D
is the dish diameter). For a
well-illuminated dish, this spacing corresponds roughly to
half-power point spacing between field centres. Because
the extent of the transform is circular, we can do somewhat better than
this, by using a so-called hexagonal grid. This grid places pointing
centres at the vertices of equilateral triangles -- packing six triangles
together gives a hexagon. An extension of Nyquist's theorem indicates that
is the wavelength, and D
is the dish diameter). For a
well-illuminated dish, this spacing corresponds roughly to
half-power point spacing between field centres. Because
the extent of the transform is circular, we can do somewhat better than
this, by using a so-called hexagonal grid. This grid places pointing
centres at the vertices of equilateral triangles -- packing six triangles
together gives a hexagon. An extension of Nyquist's theorem indicates that

So a hexagonal grid allows a given area of the sky to be covered in a smaller number of pointings (it does also require slightly longer drive times between pointings -- see below -- which may occassionally be a consideration). Table 20.1 gives this grid spacing for ATCA dishes.

Table 20.1: Mosaic grid spacing for ATCA dishes
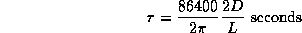
Here L
is the maximum baseline length of interest when imaging
and D
is the dish diameter.
Ideally you will want to sample twice as frequently as this, i.e. for
N
pointings, a dwell
time of 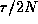 would be best. You may, however,
decide to suffer tangential holes in the u-v
coverage.
would be best. You may, however,
decide to suffer tangential holes in the u-v
coverage.
lmc_123.